Gerhard Daum: Mathematical Experimental Constructivism

A conversation with Peter Tepe | Section: Interviews

In the terminology used in w/k Gerhard Daum falls into the category of a science-related artist. He bases his work on mathematics, above all on Euclidian geometry, trigonometry and analysis. His approach is experimental, probing different techniques of mathematical construction and then reducing the constructional matrix, while continuously observing the aesthetic effect.

In addition, Gerhard Daum is one of those few people who have simultaneously done scientific and artistic work in certain development phases: in other words, he belongs to the border crossers between science and art. His interest in the laws of nature and his quest for verifiable insights mark his scientific activity. On a professional score, one of the scientific issues he has worked on concerned the development of herbal medical products.

Gerhard Daum was born in 1954 in (West) Berlin. From 1973 to 1980 his artistic preoccupation with pictorial compositions in geometric space began while he was studying chemistry and architecture in Mainz and Vienna. He went on to study for a doctorate in medicine in Berlin, followed by a period from 1988 to 2003 working in the pharmaceutical industry in the area of developing and modifying forms of medicinal drugs. From 2003 to 2014 he was engaged in hospital management in Germany and Switzerland, during which time he resumed his artistic activities. Since 2015 he has been living in Lahr in the Black Forest and devotes his time intensively to mathematically oriented constructive art.

Gerhard Daum, in our preliminary conversation you mentioned that the picture you contributed to w/k was based on a working method you typically employ. Could you please describe the most important steps in your procedure?
My working method typically starts with an elementary form of Euclidean geometry, be it a triangle, a rectangle, a pentagon, a hexagon or a spatial body (like a cube or a cuboid). I’m talking about basic elements here.

Let’s take a cube as our example. What would your next step be?
The cube with its eight vertices is projected on paper. I call them anchor points – because they are fixed.

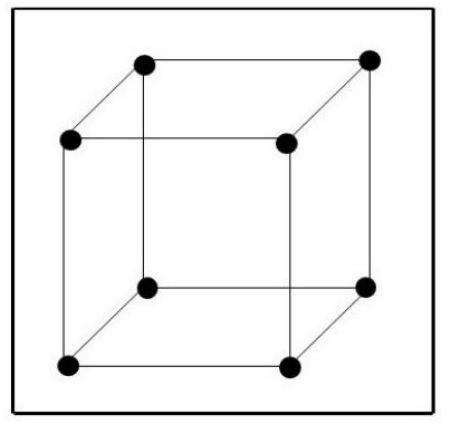
But that only gives you the starting point for a pictorial construction.
Exactly. All we have as yet is the foundation, a basic element whose anchor points form the backbone of every pictorial construction. My next step is to extend the anchor points into a kind of scaffold.

What does that look like in your example?
Very simple: I connect several anchor points with straight lines.

Which of the eight anchor points do you select?
This is where the experimental artistic work begins. To arrive at the pictorial construction in Butterfly no. 3 I plotted the straight lines in such a way as to form face and spatial diagonals inside the cube.

Could you have placed the straight lines differently?
Yes, but that would have produced a different result. And I’m not sure if I would have liked that one quite as much.

How do you continue?
I then divide each of the straight lines into equal segments. The intervals are then joined to a web of lines based on a certain correlation that already suggests the figure of a butterfly.

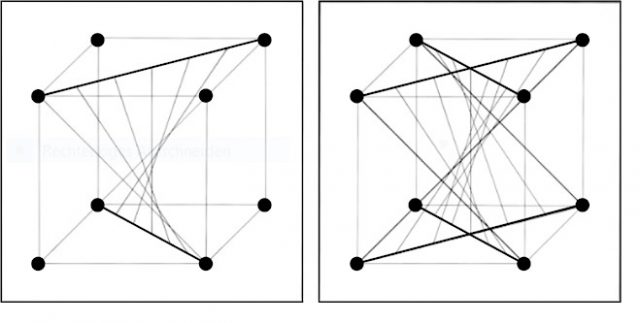
But you have to look very closely to discern a figure in this web of lines.
That’s true. And here it gets experimental and artistic again because the rigid proportions of the geometry are in a certain respect displaced and thereby modified. To do so I remove some of the straight lines or parts of them, as well as parts of the cube, in such a way that a figure – in this case a butterfly – crystallises. The basic element of the cube that initially served as a scaffold is then removed. The figure now supports itself.

So you erase lines until you arrive at a figure?
Exactly. I now do what a sculptor does with his workpiece. He chisels pieces away for as long as he needs to expose the envisaged figure in its full appearance. My finished picture emerges as the product of gradual disengagement from my initial constructional matrix which first defined the anchor points of the picture and, once this has been achieved, is then discarded in the sense of Wittgenstein’s ladder metaphor.

Is your work then completed?
As far as the artistic, creative side is concerned, yes. But now I have to transfer the construction sketch onto a sheet of drawing card measuring 50 x 65 cm that I have chosen for this purpose.

What does this involve? Is it done by tracing?
No, I resort to mathematics again. The new dimensions for the cube and the intervals have to be recalculated so that the straight lines join the anchor points and the Butterfly figure transpires as the precise result of my web of lines. Not only that, but the drawing also has to be constructed at the centre of the cardboard sheet.

Just to properly understand you, you draw the figure a second time, but now to the desired size?
Yes. Once I have calculated the dimensions I transfer the construction with pencil and ruler onto the drawing card. When I’m finished I re-draw everything with a technical ink pen using nibs between 0.18 and 1.0 mm, filling out certain areas in black. This might sound quite simple but it is actually a very complex and the most time-consuming stage of the pictorial construction since it is paramount not to draw even a single line in the wrong place. Drawing with ink pens requires the highest degree of concentration (see contribution picture Butterfly no. 3 above the article).

Besides pencil, eraser, ruler and tubular drawing pen do you use any other instruments?
I also use a protractor, and for some objects a compass too, as well as a pocket calculator to work out the dimensions of the construction

Is your typical working procedure evident to the viewer?
It is not necessary for the viewer to understand the techniques I use to achieve the aesthetic proportions. As the outcome of experimental artistic work it is the final figure itself that conveys the aesthetic experience. But in a second stage, in order to give the viewer some idea of the hidden steps leading to my pictorial construction, I also attach a kind of construction evolution to selected works, consisting of a series of sketches that clarify the work’s development and hence my work procedure.

Up till now you have explained the details of your typical artistic work process. To continue, I would like to ask about the kinds of artistic aims you are pursuing with this method. What status do harmony and beauty have in your works?
The motivation for my artistic work comes from my search for beauty, simplicity and balance. To me, the pictorial constructions are pleasing when they fulfil these criteria. And of course they should appeal to the viewer too.

How do you achieve that?
I distinguish between symmetrical and asymmetrical proportions, and aspire to connect these two principles of beauty that stand at opposite poles. First let’s look at symmetrical proportions. Through mirroring along different axes figures emerge that are characterised by order, regularity and symmetry – identical parts that add up to a whole.

That makes sense. But what I don’t yet understand is what you mean by asymmetrical proportions. Could this also give us a sense of harmony?
Yes, with asymmetrical proportions I mean the golden ratio. This is arrived at by dividing a length into two segments, whereby the smaller segment relates to the larger one in the same ratio as the larger segment does to the sum of both segments. The invariable proportion thus determined is represented by the irrational number 0.6180… In other words, a quantity that cannot be exactly measured or exactly drawn.

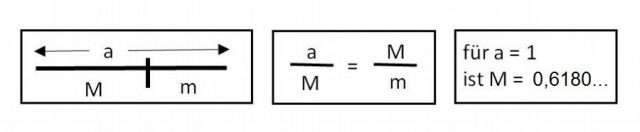
Assuming the entire length of a is 10 cm, then the largest segment M would measure 6.180… cm.

In which forms do we find these asymmetrical proportions?
Particularly fascinating is to find these proportions repeated several times within regular pentagons.

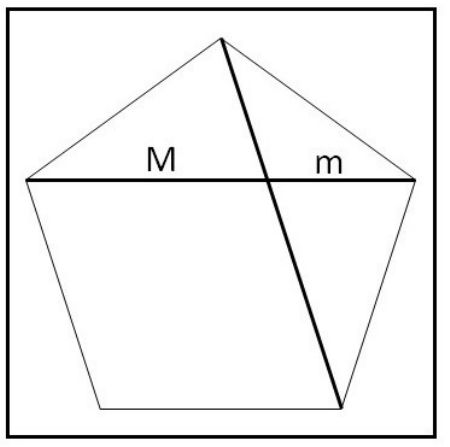
Rectangles can also be constructed in a similar manner.

With your pictorial constructions are you also interested in correspondences with the real world?
No, I am just exploring a non-representational style. Although the figure is constructed according to a mathematical calculation it doesn’t mirror reality in any experienceable way. Rather, what transpires is a figure that couldn’t be found in the real world – the grid of lines can only exist in virtual space. The harmoniously balanced form of this figure conveys an aesthetic experience, and that’s what matters to me.

Have you studied theories about beauty and harmony, and is there any theory that particularly appeals to you?
I haven’t systematically researched theories of beauty, except for incidental moments of inspiration. The artistic ideal behind my works is best described with a quote from Islamic art in around 1100. It comes from the text Kimiya al-Sa’ada (The Alchemy of Happiness) written by Al-Ghazali (1058–1111). “When any thing manifests its own potential perfection this will then be the highest level of beauty.”

Is your view of beauty and harmony tied to a certain worldview? Are you one of those artists whose concept of beauty is hinged, say, to certain underlying religious assumptions?
My view of beauty is free of religious influences; it is guided by science and mathematics. In my opinion beauty is encountered, for instance, as a lasting principle in the laws of nature, as the balance between different forms and dimensions.

Your works can clearly be described as constructive art or Constructivism. Where do you yourself see similarities here?
In the principle of mathematically and technically derived design and a non-representational style. As with other Constructivists, my constructs are preceded by planning and calculation. As for many artists in this field, elements, units of measurement and relations are precisely defined.

Did you develop your artistic programme with any particular Constructivist artists in mind who were important influences for you?
Even as a schoolboy, I was strongly impressed by the virtual worlds of the Dutch artist M. C. Escher.

What did you learn from him?
His constructions are based on geometric structures and physical laws found in nature. These range from static symmetries in manifold forms to fractals and their dynamic formation – from simple growth processes to metamorphoses. These are not then the product of human invention but things that exist independently of us. Escher’s preliminary studies show mathematical structures that can no longer be detected in the finished image. That’s how I work too. Another thing linking me to Escher is that he drew spaces on paper that couldn’t exist in the real world but nonetheless strike us as very real.

Have there been other influences from the realm of Constructivism?
Alexander Calder’s mobiles and Naum Gabo’s complex spatial constructions had an important impact on my development. With his mobiles Calder creates kinetic abstract sculptures that move within the equilibrium of asymmetry. So symmetry encompasses only one half of the world of beauty. Asymmetry enriches it with its dynamism, thereby completing the image of beauty. Gabo’s spatial constructions are evidence of a profound process of fathoming the design of sculptures in finely structured webs. This requires working with great precision, as I do too.

Are you setting new Constructivist accents through your work?
I believe that the working method I typically employ, which I described at the start of our conversation, does set new accents. But it would probably be better to ask an art historian.

Gerhard Daum, thank you for the stimulating conversation.

Further works by Mr. Daum with explanations

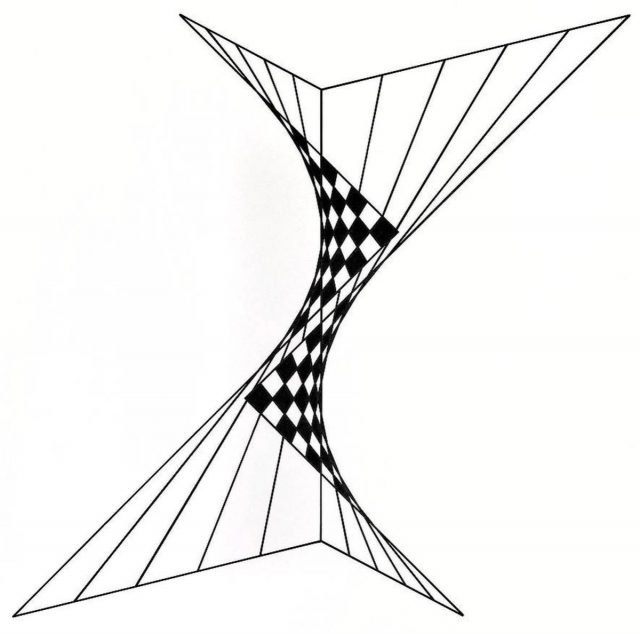
The basic element here is a cube with its eight anchor points. Diagonals are affixed to the anchor points and joined to a network of lines. After this, the straight lines and segments are removed until the figure has emerged free-standing in virtual space.

The basic element in this work is a regular pentagon whose vertices serve as anchor points for the pictorial construction. These are joined up with straight lines that are then spanned with a web of lines. Once the lines are removed the proportions of the golden ratio become apparent, revealing the internal connections of the pentagon. The ensuing result is a figure with spatial effects.

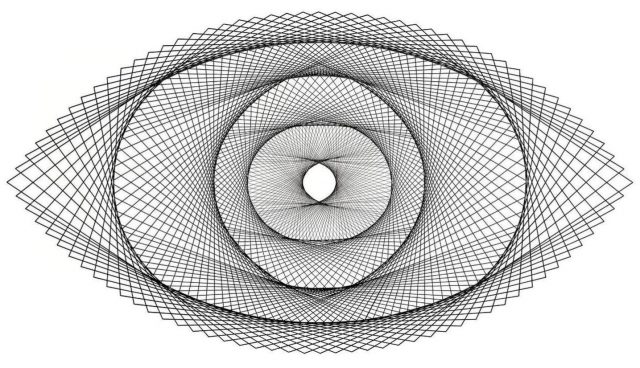
Here the primary element is a rectangle with the proportions of the golden ratio. Two circular segments are inscribed in the rectangle. These touch the median points of the rectangles’ edges. The circular segments are subdivided into intervals and joined up with lines, whose beginnings and ends are no longer visible in the finished pictorial construction. The interaction of the oval web structures generates a figure reminiscent of an eye.

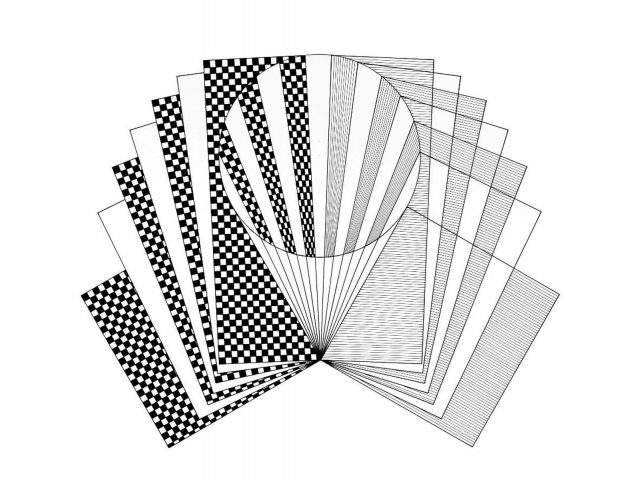
The basic element here is the secant-tangent theorem in geometry. If secants are drawn from a point outside the circle the products of the secant segments (that represent rectangles) always remain the same. It is possible to map any number of rectangles and their size, i.e. their surface area, will always be the same, while their positions, i.e. where the rectangles are located, are always predetermined. The figure follows from the given proportions, which result from the conformities to the secant theorem.

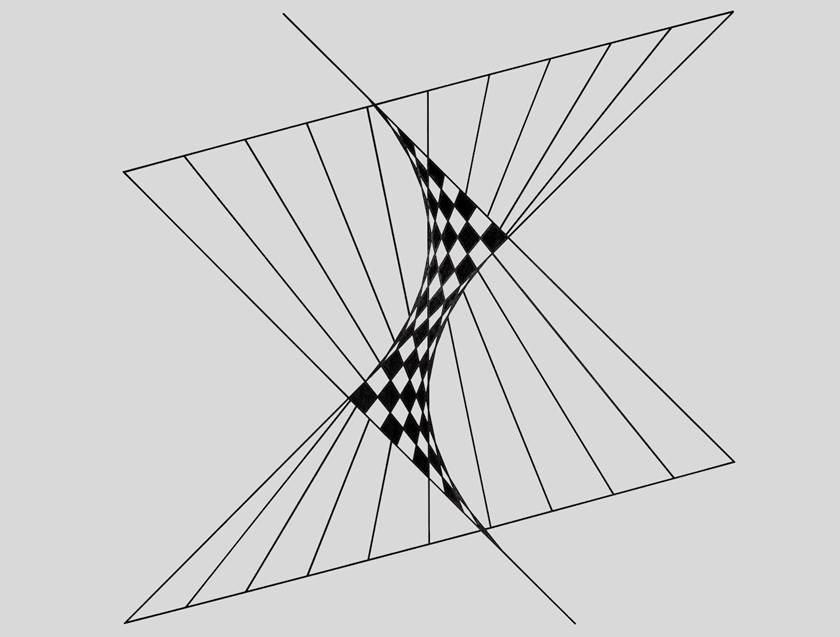
Picture above article: Gerhard Daum: Butterfly No. 3 (1976). Photo: Gerhard Daum. Tubular drawing ink pen, drawing card measuring 50 x 65 cm.

How to cite this article

Peter Tepe (2017): Gerhard Daum: Mathematical Experimental Constructivism. w/k–Between Science & Art Journal. https://doi.org/10.55597/e2369