Dominic Hopkinson: Building Blocks of Space – Mathematical Sculpting

Interview by Martin Skrodzki and Milena Damrau | Section: Interviews
Series: Mathematics and Arts

Abstract: In this conversation, Milena Damrau and Martin Skrodzki speak with Dominic Hopkinson about the role of mathematics in his artistic practice. Dominic Hopkinson generates sculptures in stone, wood, plaster, and bronze, attempting to distill these complex concepts into pure visual form. He lives in the UK.

Briefly describe your own work. Include a description of how your work relates to mathematics.
I’m a sculptor making work using various materials and techniques including stone carving, construction of wooden elements, alongside print-making, to explore how pattern and structure occur within nature.

I work collaboratively with mathematicians researching how mathematical processes can generate pattern and order from seemingly simple starting points. My main research has been into aperiodic tiling systems in multiple dimensions (i.e., into ways how to fill two-, three-, or higher-dimensional spaces with simple forms such that the resulting pattern never repeats itself); how do Penrose tiles (i.e., a pair of two tiles which provide a way to cover an arbitrarily large area in a systematic way that, again, does not quite repeat itself) and other aperiodic systems create pattern, and can this be taken into 3 dimensions? This entails understanding the connection between aperiodic tiling, X-ray crystallography (i.e., methods that experimentally determine the molecular and atomic structure of crystals using X-rays) and atomic lattices (i.e., regular, grid-like arrangements of atoms throughout a crystal), and looking at how forms can be three-dimensionally aperiodic.
Back in 2017 I received funding from Arts Council England to become Artist in Residence at the School of Mathematics, at University of Leeds. This enabled me to be embedded into a research group led by Prof. Alastair Rucklidge, alongside Dr. Priya Subramanian (Priya is now at the University of Auckland) who are studying aperiodic structures in multiple dimensions. I spent about 18 months expanding my understanding of aperiodic systems, working with the group along with PhD researchers and collaborators from different universities.
The project culminated with a piece of work being exhibited at the 2018 Venice Architecture Biennale, in a major group exhibition titled Time, Space, Existence hosted and curated by the European Cultural Centre, in Palazzo Mora, Venice.

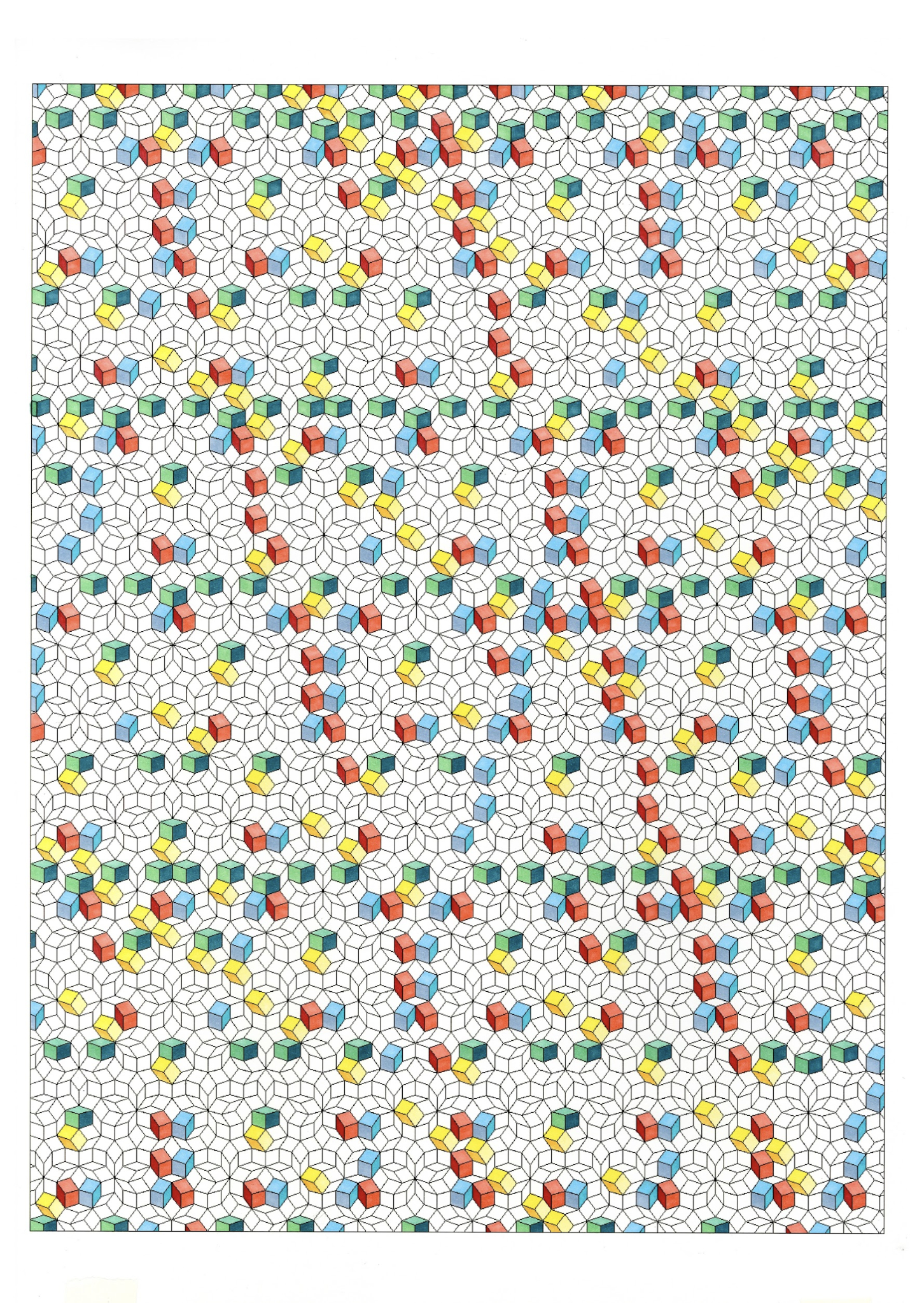
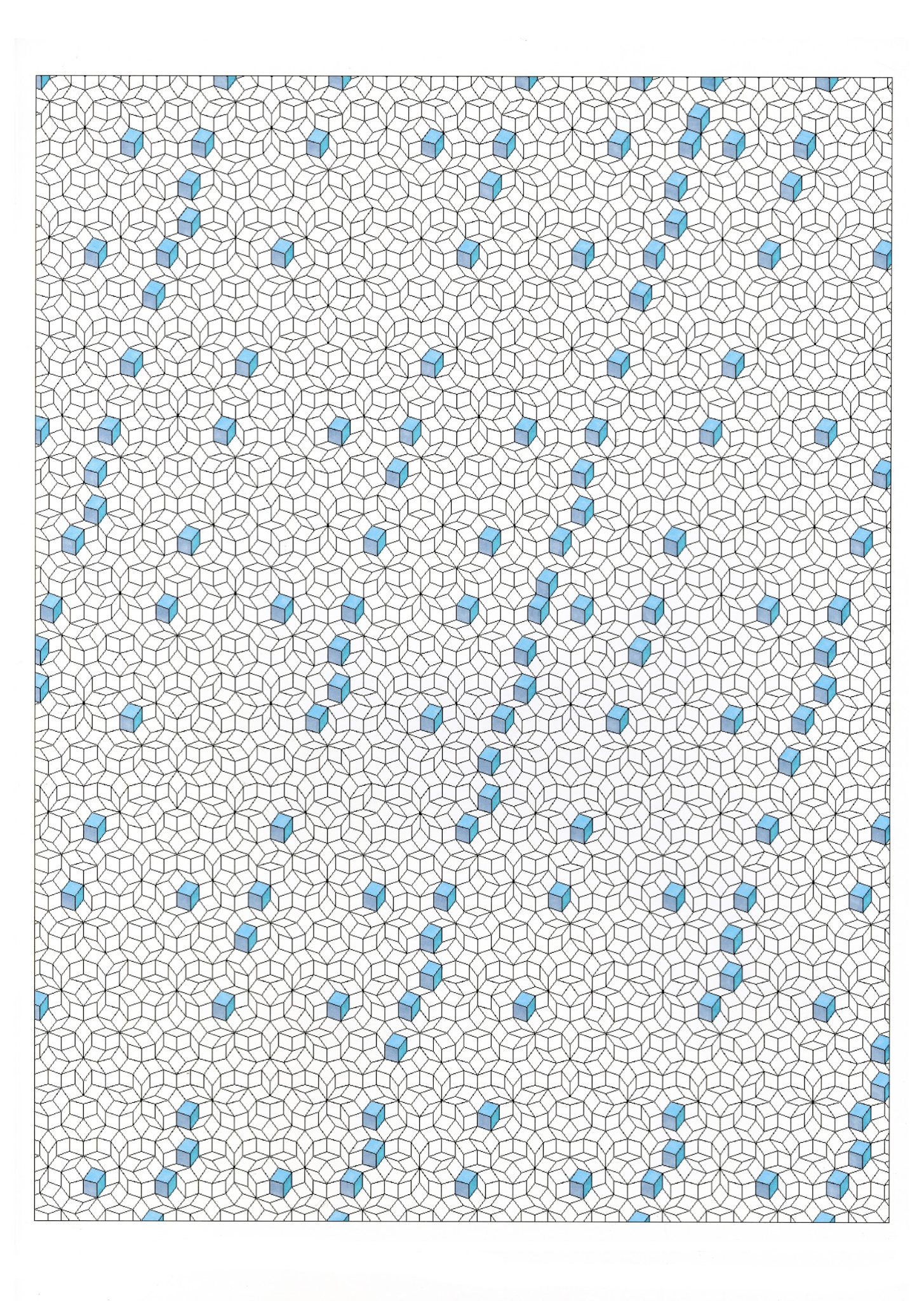
What drives/drove you to take an artistic approach to math or a mathematical approach to art? Were you always drawn to math or did you need to discover it somewhat later in your life/career?
There appears to be a set of mathematical mechanisms that define the process of how things grow, and as a sculptor an understanding of the generation of three-dimensional structure would seem to be fundamental. I study these mechanisms to learn how apparently simple mathematical systems can create complex and diverse patterns and forms in nature. These occur across scales, appearing to be similar in atomic crystal lattices as they do in cellular structures and macro objects like plants, clouds, ripples in sand and water etc.
While I was still a student studying Fine Art I read a book that changed my whole artistic and intellectual approach. This book was Chaos (J. Gleick, 2008). It opened my thinking up to very big ideas that are rooted in science and mathematics: where did we come from, what causes things to behave the way they do, what causes them to be the shape they are? At the same time I was seeing the first published large scale, colour images of fractals (i.e., ever self-repeating structures), objects that to me as a trainee artist appeared fantastical and almost other worldly, and yet such detail and complexity appears from a simple iterative equation.
I began to think of the mechanisms that cause things or objects to be the shape they are. Why are trees that shape? How do crystals grow? My earliest work was really studying what is known as closest packing theory, i.e., how do spheres fill space?

What level of mathematical knowledge did you have before you started to work on MathArt? How much of the underlying mathematics did you need to learn in order to perform your work?
I managed to just pass what was then O’level mathematics at school, aged 16. But honestly I hated the subject, wasn’t very good at it, and felt I had no instinctive understanding for the subject. Actually, it turns out that I was just very badly taught the subject at school. There was no contextualising of the subject, no early introduction to the big ideas, no explanation of the depth, utility and beauty of the subject, all things I had to discover for myself.
When I work collaboratively with mathematicians I am not attempting to learn how to solve the equations or write the code that they are working on, I’m not a mathematician. But I do want to gain a broad understanding of their processes and approach. I look for how, at a wider scale, mathematics makes connections between different ideas and other subjects. Artists tend to be very much generalists, having a bit of knowledge across a lot of interests and subjects, we make links and leaps of the imagination. I rely on this process to enable me to understand on a broad level what the mathematics is trying to do, how does the math define a system of expansion, or growth for example.
Fundamentally it seems obvious to me that mathematicians are creative people, just like artists. Their processes and ways of working are familiar; trial and error, iteration, imagination, visualisation. 
I then use this research as the basis for creating sculptural work in my studio that attempts to show the mathematical process at the same time as pointing to similarities with other processes and structures across varying scales.

Did you learn new aspects about the underlying mathematics from the process of your work?
Before I was able to work with the research group led by Prof. Alastair Rucklidge at University of Leeds School of Mathematics, I had been studying Penrose tilings and aperiodicy for a number of years in my studio. Studying, visually, the structure and order of 2-dimensional tilings by colouring in patterns and learning how those patterns were built. But after joining Alastair’s group I was able to help in analysing things like vertex arrangements of tiles (i.e., how many tiles come together at which of their tips) and translations of tiles into three-dimensional blocks that fit together following the rules of a 2-dimensional tiling.
My 18 month residency, funded by Arts Council England, at the School of Mathematics meant I was able to attend a number of international conferences about aperiodicy, quasicrystals and X-ray crystallography which gave me a much deeper appreciation of the subject.

What do you think observers of your artwork learn or gain regarding the underlying mathematics or arts? How explicit is the mathematics in your work?
Audiences are rarely specialists, in either math or art, so presenting work that is both abstract and based on mathematical theories might seem to be a challenge. Yet I am always pleasantly surprised at the responses from people who say the work is beautiful and stimulating. Viewing my work often enables audiences to become aware of this branch of math for the very first time and shows them the real connections across disparate ideas. Very few people outside of the math world have seen or heard of Penrose or aperiodic tiling, so seeing my work often enables audiences to become aware of this branch of math. 
The mathematicians I work with look at my work as representing three-dimensional atomic lattices, with each vertex (i.e., each place where tiles meet) representing a potential location for an atom in a quasicrystal lattice.
I showed my work at a math conference at ICMS Edinburgh and then saw a talk the next day about cohomology of aperiodic spaces (i.e., a method to describe and compare the shape of both low- and high-dimensional objects) in which the mathematicians quoted the work I had previously presented during their talk, which was very exciting!

In April 2022 I was invited by University of Leeds to co-present the Cheney Lecture, alongside Prof. Ron Lifshitz from University of Tel Aviv. Prof. Lifshitz gave a talk about the chemistry and physics of crystals, and I gave a talk about my work, both under the title of A Thing of Beauty: Where Science and Art Combine. The audience was socially distanced, and it was streamed online.

What new insights did other people, possibly even mathematicians, gain from your work?
One thing that surprised me was how little the connections between aperiodic patterns and fractals were known by mathematicians who don’t study aperiodicy. Many structures that are found in an aperiodic system share similarities with fractals.
The tiling patterns are also intrinsically linked to other organic patterns such as animal camouflage and ripples in water and sand etc. by a type of equation called Swift Hohenberg equations. These seem to crop up in many places in maths where systems of growth appear, and are connected to the Belousov-Zhabotinsky (BZ) chemical process that creates shifting patterns in liquids.
A general audience can see a body of work that creates connections between ideas and different processes and enables them to begin thinking about a mathematical world.

Anything else to mention?
www.dominichopkinson.com
Instagram: @dominichopkinson

References

Gleick, James (2008). Chaos: Making a new science. Penguin.

Details of the cover photo: Dominic Hopkins presenting his work for the Cheney Lecture (2022). Photo: University of Leeds.

How to cite this article

Dominic Hopkinson, Martin Skrodzki and Milena Damrau (2023): Dominic Hopkinson: Building Blocks of Space – Mathematical Sculpting. w/k–Between Science & Art Journal. https://doi.org/10.55597/e8862